END OF AN ERA, FRACTALFORUMS.COM IS CONTINUED ON FRACTALFORUMS.ORG

Wow, beautiful pirctures! Nice work, all. I might like the non-conformal version slightly better, with it's angular IFS shapes, but this one is interesting too.
DarkBeam, is your seahorse picture from the variant you created? Your variant uses the same conformal map as Tglad's transform, it just uses a different projection onto the sphere, is that correct?

Knighty: I haven't checked the code, but the stereographic projection should not affect the formula or the shape, it should just be a more economical way to go from the complex plane to the sphere, and back again.


You are right, that is what I was doing in the two links at the start of this topic. I didn't have an analytic formula so I was doing it using a lookup table. It would make the formula 'quasi-conformal' in that the stretch would be bounded (maximum eccentricity of 2 I think), however it would make the formula non-smooth at those 6 points.
(I'm fairly sure an analytic formula could be be made using a weighted average of conical transforms at those 6 points, and 3 poles and 4 points that go to zero but scale by about 2. The weighting being something like 1/distance to feature, on the sphere).

Exactly conformal is impossible due to Liouville's theorem, since the only conformal 3d transforms are translations, rotations, dilation (scale) and sphere inversions, and combinations of these. The same is true in hyperbolic space and in dimensions more than 3d. But *nearly* conformal (quasiconformal) is an interesting question, I got an average eccentricity of about 1.5 in this post- http://www.fractalforums.com/the-3d-mandelbulb/a-new-3d-mandelbrot-like-fractal/msg12013/#msg12013

My previous 2d mandelbrots were wrong (I was adding c in the numerator). I have also written a more correct bailout condition and the results are very cool:

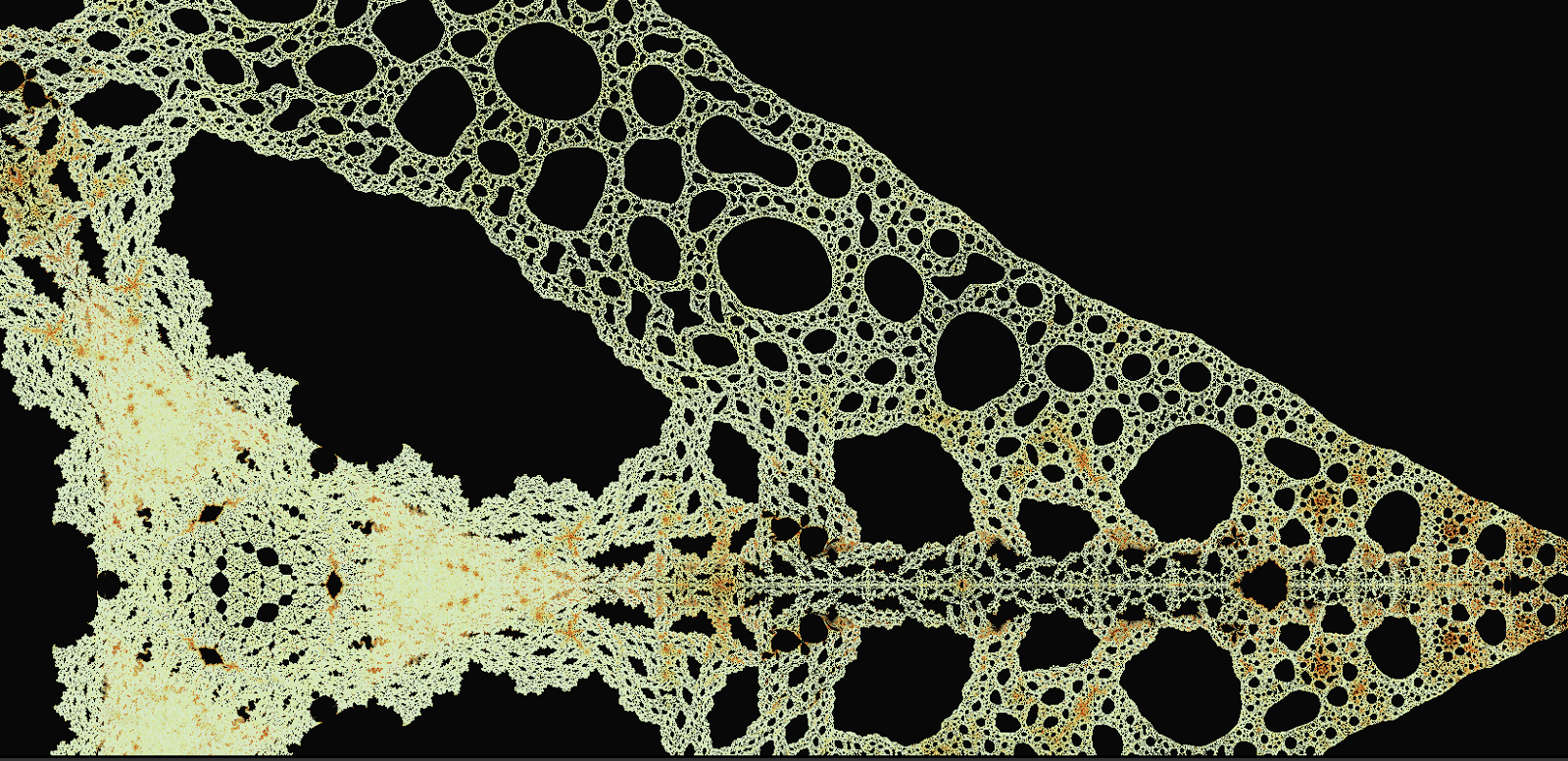
(the full shape is a rough triangle)


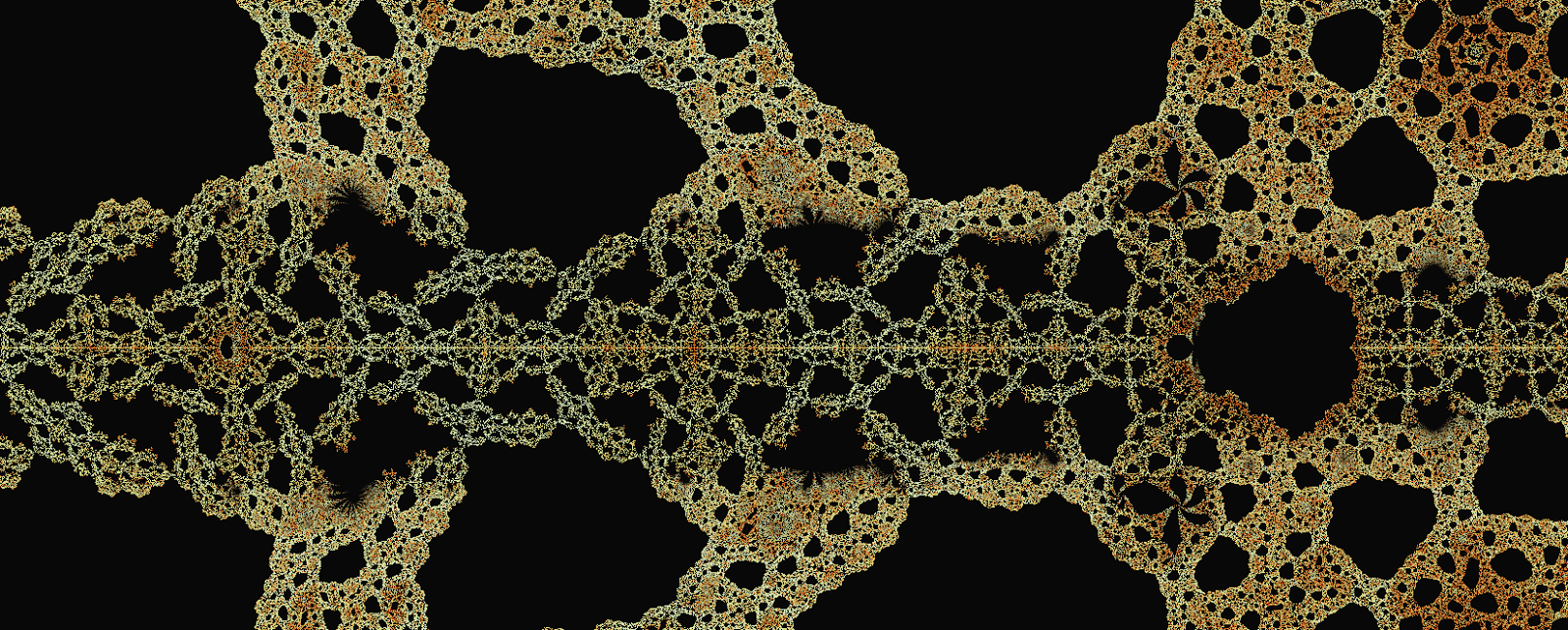
and for the dihedral fold version:




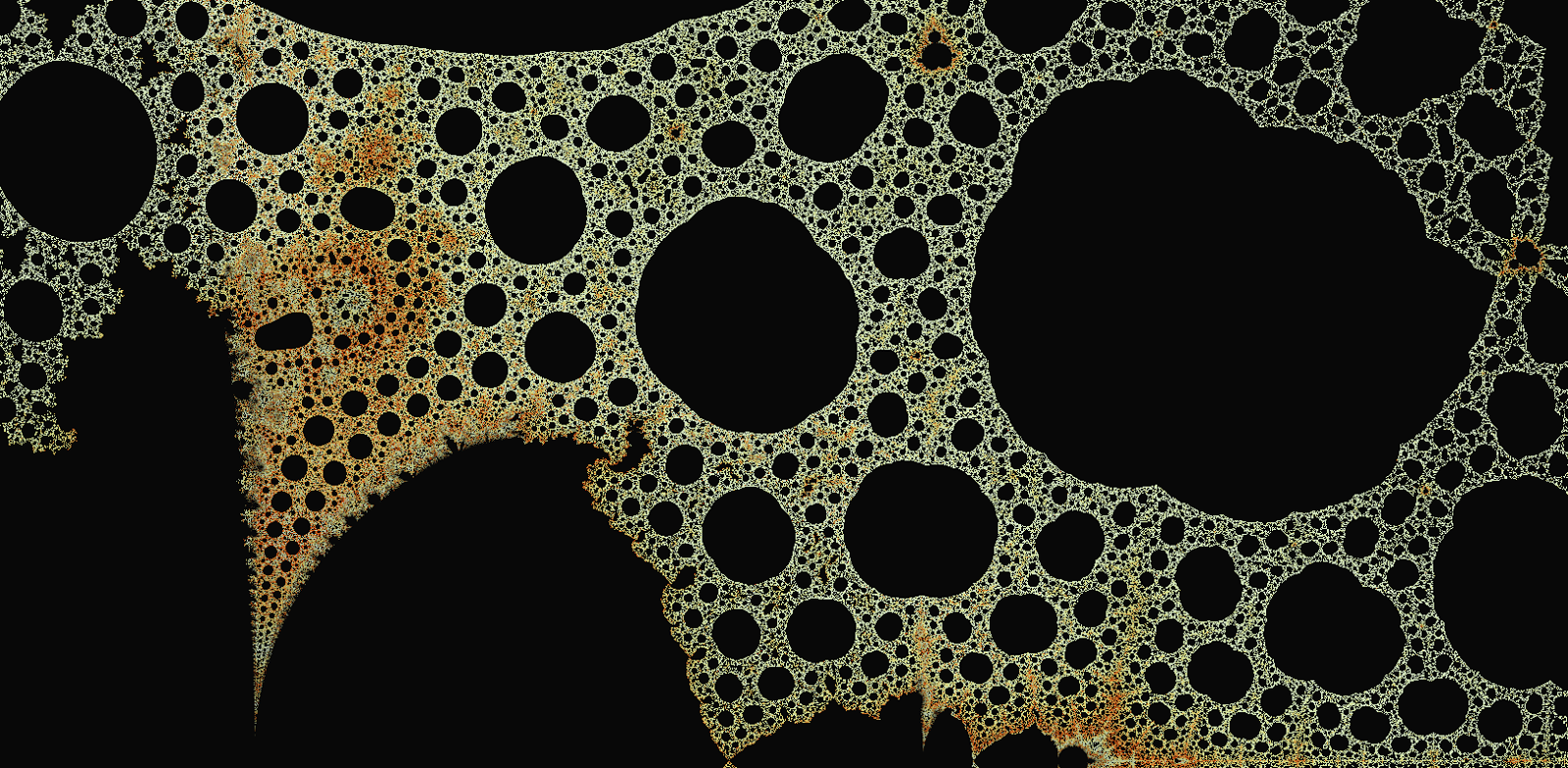
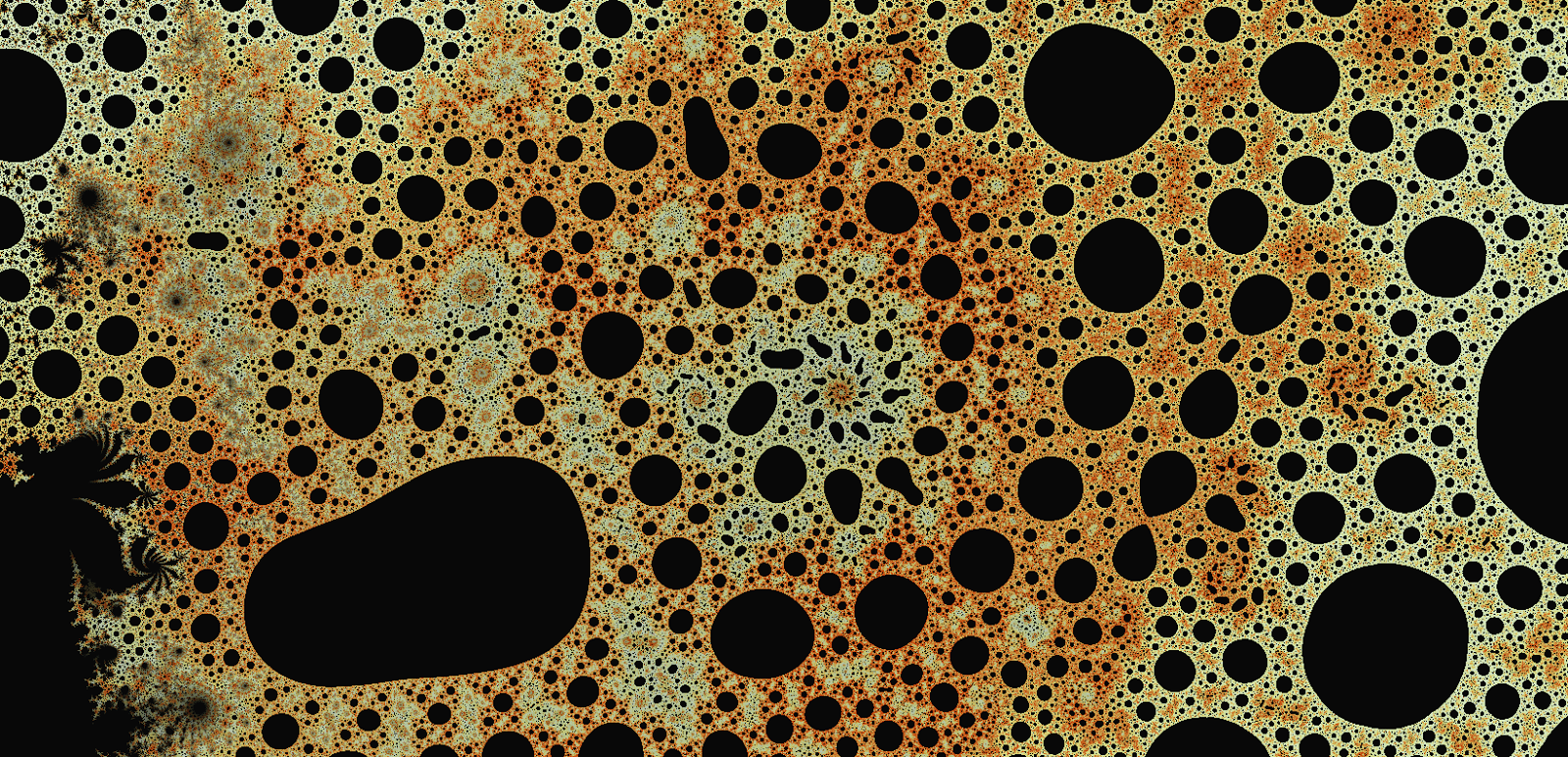
Try it out, I saved it here: http://hirnsohle.de/test/fractalLab/ under 'Tetrahedral fold' in the library. You can change the fractal type to Dihedral in the constants tab.

Tglad & DarkBeam:
Ah! yes, the stereographic projection gives the same result. It's only rotated 180°. to get exactly the same result just set:
vec2 z = - p.xy / (1. - p.z);
Instead of:
vec2 z = p.xy / (1. - p.z);
in the beginning of  tetra() function.
So no need for trigonometric functions.

I didn't know (and couldn't find docs about it) that liouville's theorem holds also in 3D+ hyperbolic spaces. Any references?

The existence of points where the derivative of tetra() vanishes may explain why the julia of this fractal contains (a little bit deformed) 2D classic julias.

Nice Julia stone!
from here: http://en.wikipedia.org/wiki/Liouville%27s_theorem_(conformal_mappings)
I think it is basically expressed in this sentence:
"Similar rigidity results (in the smooth case) hold on any conformal manifold. The group of conformal isometries of an n-dimensional conformal Riemannian manifold always has dimension that cannot exceed that of the full conformal group SO(n+1,1)."    (Hyperbolic space being one such conformal Riemannian manifold).
In other words, if the manifold is 3d, then the conformal symmetries are just SO(4,1) (4d rotations) which is 6 dimensional which I'm fairly sure is seen as the combinations of translation, rotation, inversion, dilation in 3d.
Some more info here: http://en.wikipedia.org/wiki/Conformal_geometry#Higher_dimensions. All in horribly obscure language of course. I think this also suggests the same is true in Minkowski space.

I have attached the fractalLab code so you can use it (apparently saving to the library is only local).
The negative versions of both formula are equally awesome, if not more so-

  I was finally able to drop all trigonometric funx to Tglad's brot:

Math has its own technical language. There are tons and tons of words which mean very simple things but if you had to explain them each time again in full sentences (i.e. you'd not already have a word for that), you'd end up talking for hour where a minute would have sufficed.
Really, any specialized field has these same issues. I wish there was a way around that but I fear there isn't really any.

In fact that's probably a big part of learning new stuff: Find new ways to express it. Learning something deeply means being able to talk about it fluently.

This is going to be another busy weekend...