END OF AN ERA, FRACTALFORUMS.COM IS CONTINUED ON FRACTALFORUMS.ORG

To what I've learned through intuition, exploration and logic:
the number of infinities per bulb is multiplied by the periodnumber of the bulb.
The maincardiod is infinity*1 , the main bulb (which i find is a misleading name) is infinity*2 and so on.

I don't know about the circumference.

That's a coincidence. It occurred to me yesterday that the number of disks has to be countably infinite. Between any two given areas there can only be finitely many disks that have such area, otherwise the mandelbrot set would have inifinite area. That means we can speak of a largest disk, a second largest disk etc. Sorting the disks in that order gives a countably infinite list:
first those with area between some upper bound of the mandelbrot set area and 1/2 the upper bound
then those between 1/2 and 1/4
then between 1/4 and 1/8
...

I think it's insightful because the reasoning also works for other situations where there's an infinite number of surface pieces that each have positive area while it is known that their sum has finite area. I guess this is trivial for people more into mathematics (unless it's wrong xD)


I don't know what you mean by a ratio of infinities. How would you compare those? 2*infinity isn't really different from 1*infinity. If you slice the mandelbrot set in 2 parts, both parts will contain minibrots which are identical to the whole set, so the length of the circumference is the same infinity I guess.

wow, i really enjoyed those fantastic comments!!!

If i say my opinion on this i will probably confuse everyone into sanitorium avoidance mode. because i dream a lot and my understanding of maths and physics is comical.   So here is the theory:

The reason why i asked is because i became very confused about physics some time ago to understand what happens beyond schwartzchild radius. Programming synthesizers and learning cymatics emulator theory candidly convinced me that the probabilistic density of wave motions and therefore the wave number compared to the input must increase inside black holes when waves fold over each other into infinitely smaller states of stasis in different compression gradients and that it creates new universes and it creates any kinds of timespace and dimensions and universes imaginable. when one wave is frequency modulated by another it produces exponentially more information than additivesly, same with orbit of 4 space entities being more complex than 2 non folded orbits of 2 entities. this theory helped me to overcome the confusion about thinking about infinite gravity.

so the universe would be biologically self replicating which is a good basis for existence of something. it's aliiive .

The mandelbrot set has something similar to the "black hole and white hole" theory, where the division between bulbs can represent the place when a black hole goes into a white hole and where the number of Cosmic backgroud radiation distances in the total universe is more numerous than the atoms in the ocean, so we consider if it is isotropic if we travel many CMBR radius away i.e. a gogleplex. That's cool because the infinities spiral off into more infinities in the mandelbrot, and for me that's what's a black hole must be like.

So after i saw a figure of potato baby/figure of 8 fractal that i've seen in various places that have always confused me which can happen with video echo, and i compared the bulbs to a mandelbrot because they have a similarity. :
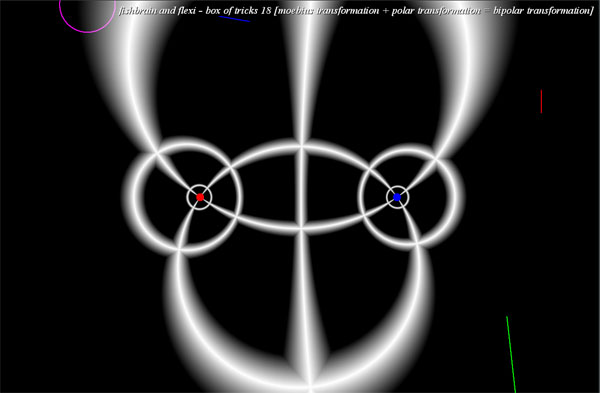
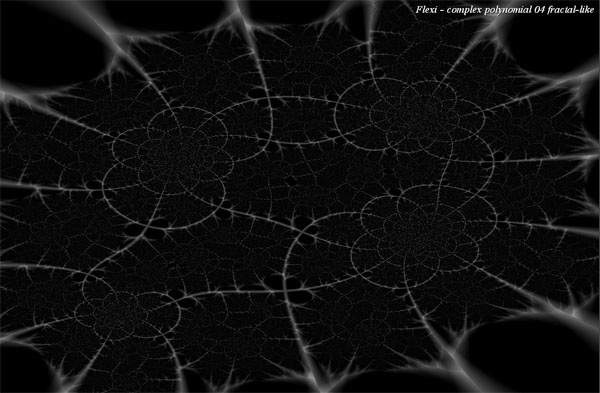
This is similar to the mandelbrot to me because there are two major bulbs on it. So i figured, what's the ratio of infiniti on the different sides of the mandelbrot... if there can be as many CMBR distances as there are atoms in the ocean i figured i should get my head round it but i failed. thanks.