The All New FractalForums is now in Public Beta Testing! Visit FractalForums.org and check it out!
|
|
|
||||||
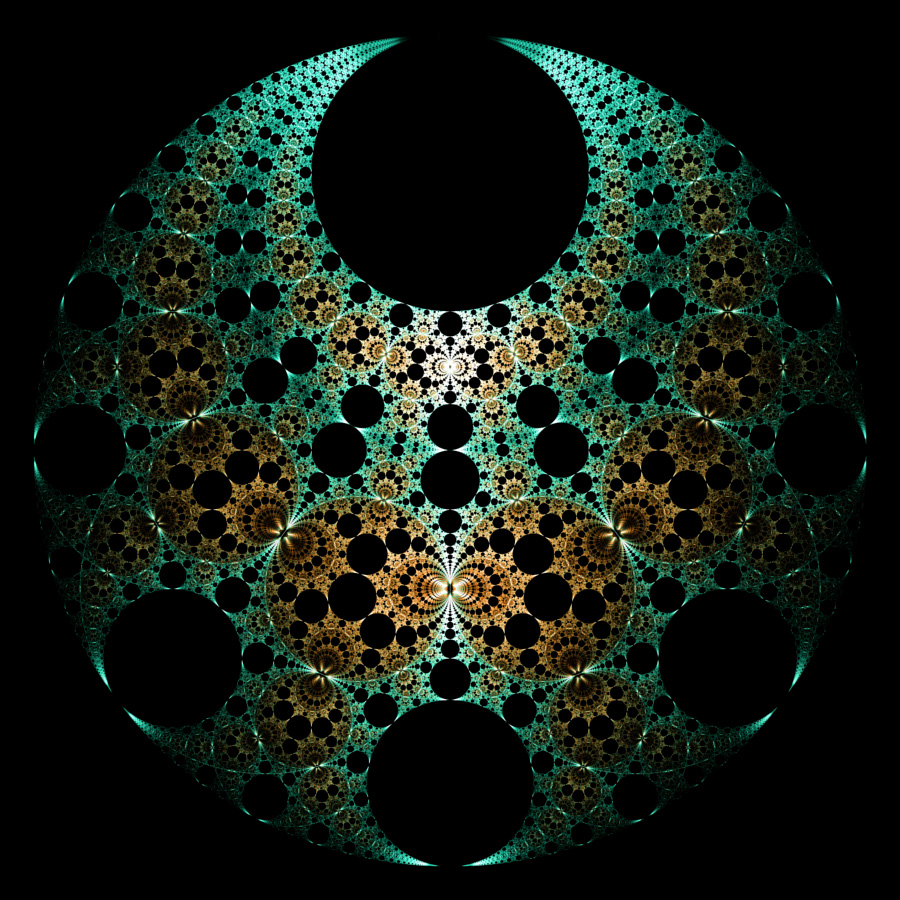
| Egyptian Necklace | ||||||
| Previous Image | Next Image | ||||||
| Description: Created with the Fractal Science Kit fractal generator. See http://www.fractalsciencekit.com/tutorial/examples/examples.htm for details. Stats: Total Favorities: 0 View Who Favorited Filesize: 412.15kB Height: 900 Width: 900 Discussion Topic: View Topic Keywords: fractal science kit attractor IFS mobius Posted by: Ross Hilbert  November 18, 2010, 01:34:08 PM November 18, 2010, 01:34:08 PMRating:      by 3 members. by 3 members.Image Linking Codes
|
||||||
| 0 Members and 1 Guest are viewing this picture. | ||||||
| Related Images | ||||||
|
|
Comments (2)  |
|
| Ross Hilbert | November 18, 2010, 03:22:02 PM The basic idea is that you define an IFS from a set of Mobius transformations. The Mobius transformations I used for this image are: Mobius m1 = Mobius(1, 0, -1i, 1) Mobius m2 = Mobius(1, -1, 0, 1) Mobius m3 = Mobius(-1, 0, 0, 1) Mobius m4 = Mobius(1, -1-2i, 0, 1) Mobius m5 = Mobius(1, 1-2i, 0, 1) Mobius i1 = Mobius.Inverse(m1) Mobius i2 = Mobius.Inverse(m2) Mobius i3 = Mobius.Inverse(m3) Mobius i4 = Mobius.Inverse(m4) Mobius i5 = Mobius.Inverse(m5) Ross |
| Bent-Winged Angel | November 18, 2010, 02:41:46 PM Well done! I really like this style that you do. However I do not see the "mobius" part. Care to exp[lain? IE: Istarted a thread in the "let's collaborate" section on mobiusa. Hope to learn more!  |
Powered by SMF Gallery Pro