The All New FractalForums is now in Public Beta Testing! Visit FractalForums.org and check it out!
|
|
|
||||||
| Silken Siegels | ||||||
| Previous Image | Next Image | ||||||
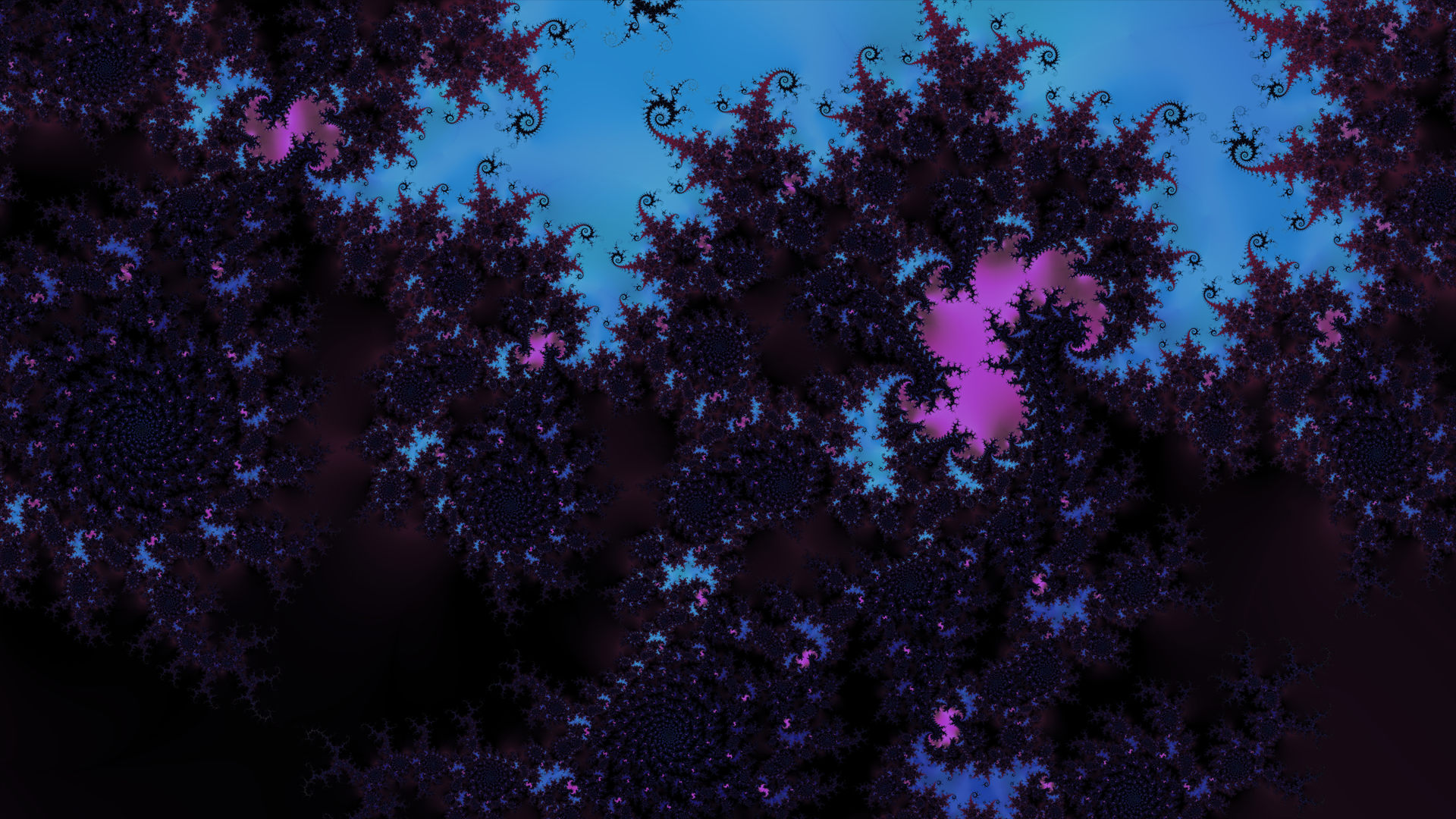
| Description: A Triskelion marriage of three different quadratic Siegel Disk basins -- the corresponding z2 Julia seeds are on the boundary of the M-set cardioid, at three different points on that boundary. The spirals "in between" the disk basins are evidence of another class of repelling periodic points with the potential to condense into a fourth basin given somewhat different parameters -- i.e., there's really another Julia set, a disconnected one, lurking here. Of course, every point not in the interior of any of the basins is somehow a part of all five Julia sets -- the three quadratic Siegel disk basin boundaries, the disconnected one, and the Triskelion Julia set. Julia sets within Julia sets ... as with the spacefillers, where one somehow has multiple cryptic disconnected quadratic Julia sets each one of which is the whole Riemann sphere, yet which seem to "interlock" to sum up to the whole Riemann sphere at the same time, it's clear that some new mathematics is needed to describe aspects of what goes on here. The colorings that render the spacefillers' structures visible suggest it may be a form of fuzzy-set that's relevant -- each point can belong "strongly" versus "more weakly" to each of the combined subordinate Julia sets, that belongs in non-fuzzy-set terms to all of them by belonging to the parent Triskelion (or Matchmaker) Julia set (whether or not the latter is the whole Riemann sphere, as in the spacefilling case, or a proper subset, as in this image). Of course, one might argue that the spacefillers' inner structures are illusory -- after all, it's really just the entire sphere! -- yet the fact that picking a seed in the butt crack of one minibrot and near the antenna of a second results in a spacefiller where the relevant colorings all show Elephant Valley shapes intertwined with linear ones establishes firmly that what these colorings bring out is real, reflecting some actual way in which the dynamics of the individual quadratic Julia sets are sub-dynamics of the dynamics of the spacefilling Julia set. So, the subordinate Julia sets "really are in there" in some sense, yet also every point in the Riemann sphere belongs to all of them, so the way to describe how they are "in there" cannot be the simple binary membership language of "normal" set theory. Instead it must be some measure, perhaps a simple scalar or perhaps more than that, which colorings like weighted-sums, Elliptic Harlequin, triangle inequality average, and others can in some manner approximate. Stats: Total Favorities: 0 View Who Favorited Filesize: 492.91kB Height: 1080 Width: 1920 Discussion Topic: View Topic Keywords: Triskelion Posted by: Pauldelbrot  April 06, 2014, 06:17:49 AM April 06, 2014, 06:17:49 AMRating: Has not been rated yet. Image Linking Codes
|
||||||
| 0 Members and 1 Guest are viewing this picture. |
|
Comments (0)  |
Powered by SMF Gallery Pro